The Mathematics of the Great Pyramid
The Queen's Chamber Passage
The most unusual aspect of the passage is the side walls from the north wall of the gallery to 771 plus inches down the passage. Each side is made up of pairs of stones 2 cubits long, one on top of the other, that have their vertical joints in line with each other, something not done anywhere in the pyramid or really anywhere else in architecture because it lacks the structural strength that overlapping has. At the south end of this arrangement are two sets of longer stones measuring 72 inches. It is mentioned that the pairs are almost the same height, which probably means that they are the same height but the floor is inserted between the walls, obscuring the rest of the block. Maragioglio and Renaldi show 19 sets on the east side plus the two longer ones in their drawing. If the longer ones were divided, they would total 23, the number of chromosomes we inherit from each of our parents, but that would be a huge stretch. The west side has fewer stones because of the exit space of the Well Shaft.
With the appearance of all the 7’s associated with the passage and the Queen’s Chamber, the length of these pairs might well be 771.6049381, whose square root is 27.7777777. 771.6040064, a difference of only .000917,
This length divided by 17 becomes 45.3884709, an eighth of 363.1077677, which, divided by 100, is the ratio between First Line and Third Line in the Golden Proportion world view.
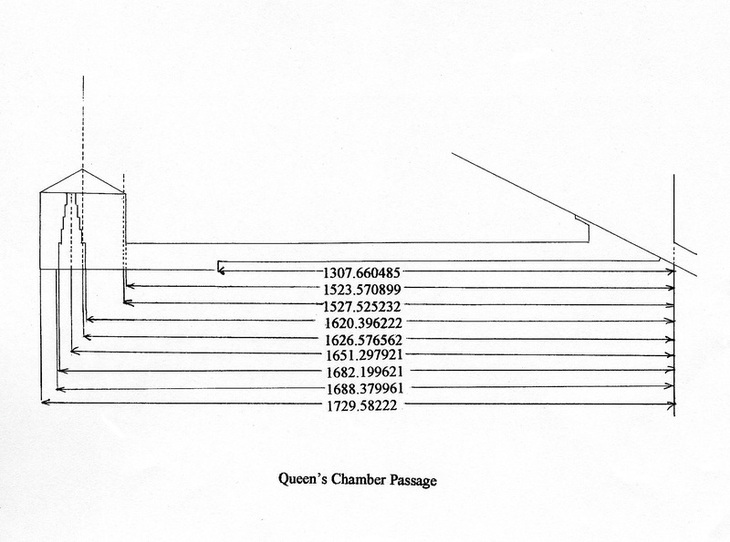
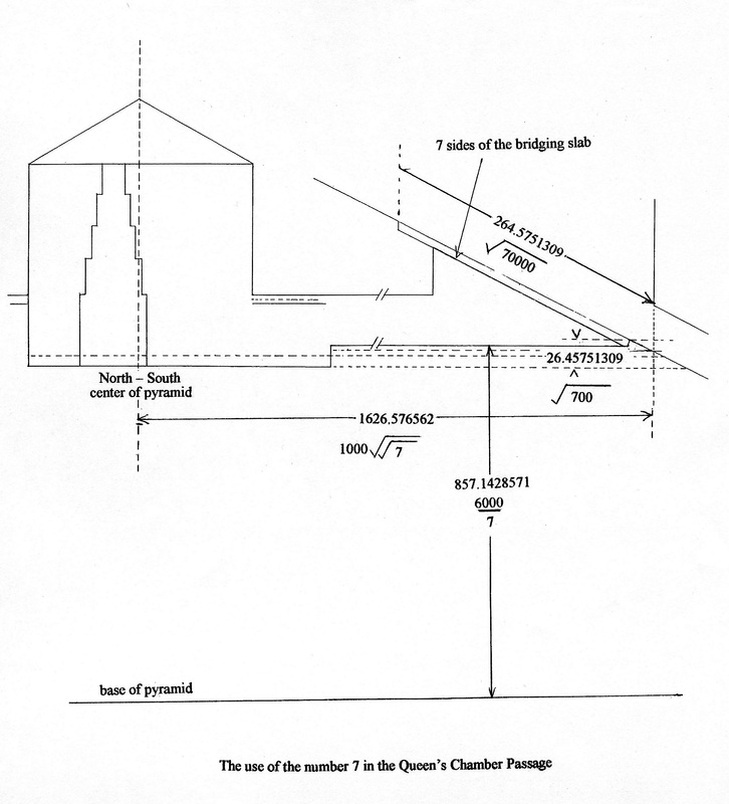
The length of the passage from the Grand Gallery‘s north wall up to the drop in the floor is 1307.004294 inches, and to the chamber entrance, 1523.570897, which is obtained by subtracting 103.0056648 (half of the chamber’s width of 206.0113296 inches) from the 1626.576562 inches length of the passage to the chamber’s center. Although this is close to 261.803988, there is another measurement that may take precedence and that is from the down step to what is called Smythe’s floor joint number 30, which is just inside the chamber. This is 220.520939 inches. Its inverse is 4538.847096, an eighth of the pyramid’s perimeter of 36277.73439 inches.
This would make Smythe’s joint number 30, 1527.525231 inches from the start of the passage. 1527.525231 is the square root of 2333333.3333. The inverse of 2.333333333 is 42857412857, 7’s repeating digits. When 1527.525231 is multiplied by 7 it becomes 3 to the 10th power. Divided by the square root of 7 it becomes 5773502687, the Third Line‘s ratio with Sixth line and also the height of the pyramid. This ratio divided by 1.023334547, (the ratio between 35.77708764 and 3661192971) is the square root of the inverse of the Golden Proportion’s ratio between First Line and Second Line, 3.141640789, the pyramid’s inside pi. 1.527525231 is also the ratio between the square root of 7 and the square root of 3, giving 7 and Seventh Line, even more say in the scheme of things.
With the appearance of all the 7’s associated with the passage and the Queen’s Chamber, the length of these pairs might well be 771.6049381, whose square root is 27.7777777. 771.6040064, a difference of only .000917,
This length divided by 17 becomes 45.3884709, an eighth of 363.1077677, which, divided by 100, is the ratio between First Line and Third Line in the Golden Proportion world view.
The length of the passage from the Grand Gallery‘s north wall up to the drop in the floor is 1307.004294 inches, and to the chamber entrance, 1523.570897, which is obtained by subtracting 103.0056648 (half of the chamber’s width of 206.0113296 inches) from the 1626.576562 inches length of the passage to the chamber’s center. Although this is close to 261.803988, there is another measurement that may take precedence and that is from the down step to what is called Smythe’s floor joint number 30, which is just inside the chamber. This is 220.520939 inches. Its inverse is 4538.847096, an eighth of the pyramid’s perimeter of 36277.73439 inches.
This would make Smythe’s joint number 30, 1527.525231 inches from the start of the passage. 1527.525231 is the square root of 2333333.3333. The inverse of 2.333333333 is 42857412857, 7’s repeating digits. When 1527.525231 is multiplied by 7 it becomes 3 to the 10th power. Divided by the square root of 7 it becomes 5773502687, the Third Line‘s ratio with Sixth line and also the height of the pyramid. This ratio divided by 1.023334547, (the ratio between 35.77708764 and 3661192971) is the square root of the inverse of the Golden Proportion’s ratio between First Line and Second Line, 3.141640789, the pyramid’s inside pi. 1.527525231 is also the ratio between the square root of 7 and the square root of 3, giving 7 and Seventh Line, even more say in the scheme of things.
page 33